Understanding The Arclength Formula Integral: A Comprehensive Guide

The arclength formula integral is a crucial concept in calculus and geometry, helping us measure the distance along a curve. This formula plays an essential role in various fields, including physics, engineering, and computer graphics. Understanding how to derive and apply the arclength formula integral can enhance your mathematical skills and problem-solving abilities. In this article, we will explore the arclength formula integral in detail, providing insights, examples, and applications that will deepen your comprehension of this vital topic.

Calculating the length of a curve is not just a theoretical exercise; it has practical applications in real-world scenarios. For instance, engineers often need to calculate the lengths of paths for roads or cables, while architects rely on it to design structures that follow specific curves. By mastering the arclength formula integral, you can tackle such challenges with confidence.

This article will guide you through the derivation of the arclength formula integral, its applications, and examples to illustrate its use. Whether you're a student, educator, or professional, this comprehensive overview aims to enhance your understanding of this important mathematical concept.

Table of Contents

• 1. Introduction to Arclength
• 2. The Arclength Formula Integral
• 3. Derivation of the Arclength Formula
• 4. Applications of the Arclength Formula
• 5. Examples of Arclength Calculations
• 6. Common Mistakes in Arclength Calculations
• 7. Advanced Topics in Arclength
• 8. Conclusion

1. Introduction to Arclength

Arclength refers to the distance along a curve between two points. Unlike a straight line, measuring the length of a curve requires a different approach due to its geometric properties. The concept of arclength is fundamental in both mathematics and physics, as it allows for a deeper understanding of curves and their properties.

In a two-dimensional space, a curve can be defined parametrically or as a function. The arclength of a curve can be determined using specific integral calculations, which we will delve into later in this article. Understanding arclength is essential for anyone studying calculus, as it provides insights into the behavior of functions and their graphical representations.

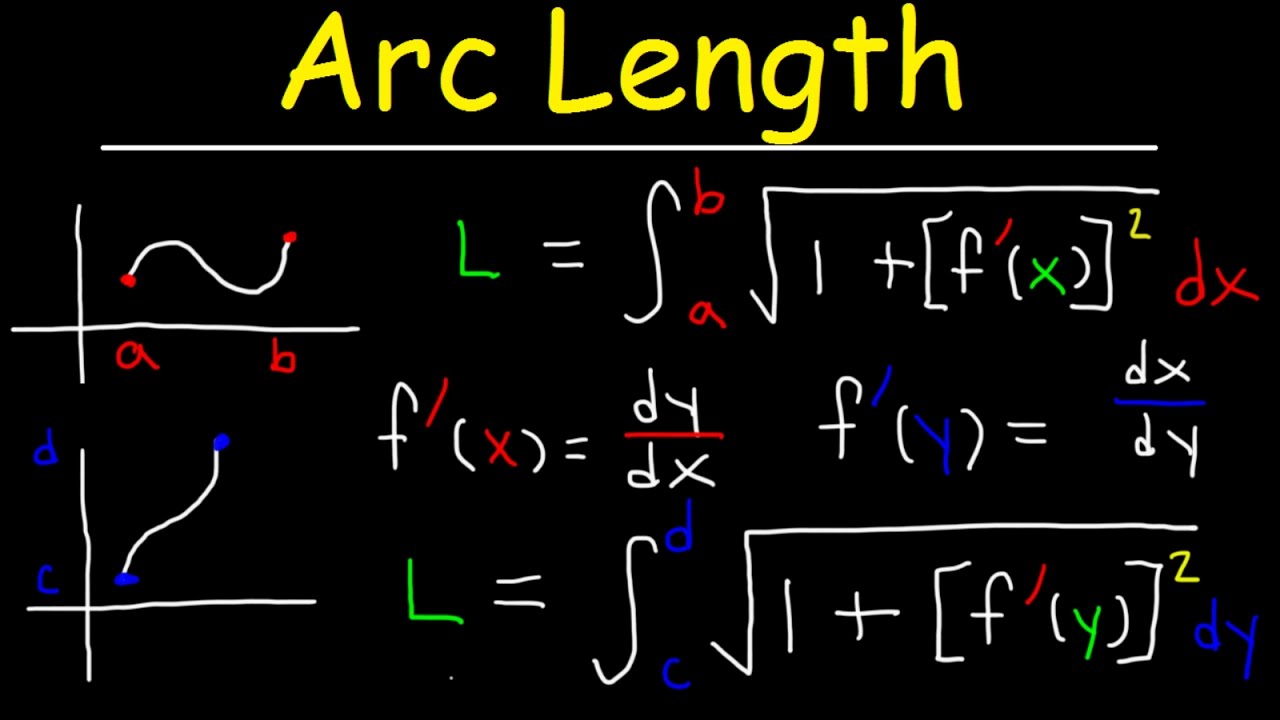
2. The Arclength Formula Integral

The arclength formula integral is expressed mathematically as follows:

L = ∫_a^b √(1 + (dy/dx)²) dx

In this formula:

• L represents the total arclength of the curve.
• a and b are the limits of integration, which define the interval over which we are measuring the arclength.
• dy/dx is the derivative of the function defining the curve.

This formula provides a way to calculate the length of a curve by integrating the square root of the sum of 1 and the square of the derivative of the function. It is applicable for curves defined by continuous functions over a specified interval.

3. Derivation of the Arclength Formula

To derive the arclength formula integral, we start with the concept of a small segment of the curve. Consider a curve defined by the function y = f(x) between the points (a, f(a)) and (b, f(b)). We can approximate the length of this curve by breaking it into small linear segments.

For a small segment of the curve, the length can be approximated using the Pythagorean theorem:

ds = √(dx² + dy²)

Here, ds represents the differential length of the curve. By expressing dy in terms of dx using the derivative, we can rewrite this as:

ds = √(dx² + (dy/dx)² dx²) = √(1 + (dy/dx)²) dx

To find the total arclength, we integrate this expression over the interval from a to b:

L = ∫_a^b √(1 + (dy/dx)²) dx

4. Applications of the Arclength Formula

The arclength formula integral has numerous applications across various fields:

• Engineering: Used to calculate lengths of curved structures, such as bridges and roads.
• Physics: Essential in understanding motion along curved paths and trajectories.
• Computer Graphics: Helps in rendering curves and paths accurately in visual designs.
• Architecture: Assists in designing buildings with curved facades and structures.

5. Examples of Arclength Calculations

Example 1: Arclength of a Simple Curve

Consider the curve defined by the function y = x² from x = 0 to x = 1. To find the arclength, we first calculate the derivative:

dy/dx = 2x

Now we substitute this into the arclength formula:

L = ∫₀¹ √(1 + (2x)²) dx = ∫₀¹ √(1 + 4x²) dx

Evaluating this integral will give the total arclength of the curve.

Example 2: Arclength of a Parametric Curve

For a parametric curve defined by x(t) = cos(t) and y(t) = sin(t) for t from 0 to 2π, we can use the following formula:

L = ∫₀^2π √((dx/dt)² + (dy/dt)²) dt

Calculating this will yield the arclength of the circular path.

6. Common Mistakes in Arclength Calculations

When calculating arclength, several common mistakes can occur:

• Neglecting to take the derivative correctly.
• Forgetting to adjust the limits of integration for parametric equations.
• Failing to simplify the integrand before integration.

Being aware of these pitfalls can help you avoid errors in your calculations.

7. Advanced Topics in Arclength

As you become more comfortable with the arclength formula integral, you may encounter advanced topics, such as:

• Arclength on surfaces in three-dimensional space.
• Calculating arclength for vector-valued functions.
• Applications of arclength in differential geometry.

Exploring these advanced topics can enhance your understanding and ability to apply arclength calculations in more complex scenarios.

8. Conclusion

In conclusion, the arclength formula integral is a valuable tool in mathematics, allowing us to measure the distance along curves with precision. By understanding its derivation, applications, and common pitfalls, you can effectively utilize this formula in various fields. We encourage you to explore further examples and applications to solidify your comprehension of arclength.

If you found this article helpful, please leave a comment below, share it with your friends, or explore other articles on our site for more mathematical insights.

Thank you for reading, and we hope to see you back for more engaging content!