How To Solve Quadratic Inequalities: A Comprehensive Guide

Quadratic inequalities are mathematical expressions that involve a quadratic function and an inequality symbol. Understanding how to solve these inequalities is essential for students and anyone interested in mathematics. This guide will take you through the step-by-step process of solving quadratic inequalities, providing you with the tools necessary to tackle these problems confidently.

In this article, we will explore the concept of quadratic inequalities, the methods used to solve them, and various examples that illustrate the process. Whether you are preparing for an exam or simply looking to enhance your math skills, mastering quadratic inequalities is crucial for your mathematical journey.

We will also delve into the importance of quadratic inequalities in real-world applications and why having a solid grasp of this topic can be beneficial. So, let’s dive into the world of quadratic inequalities and discover how to solve them effectively!

Table of Contents

• Understanding Quadratic Inequalities
• Basic Terminology
• Methods to Solve Quadratic Inequalities
• Graphical Method
• Algebraic Method
• Example Problems
• Real-World Applications
• Common Mistakes
• Tips for Solving Quadratic Inequalities
• Conclusion

Understanding Quadratic Inequalities

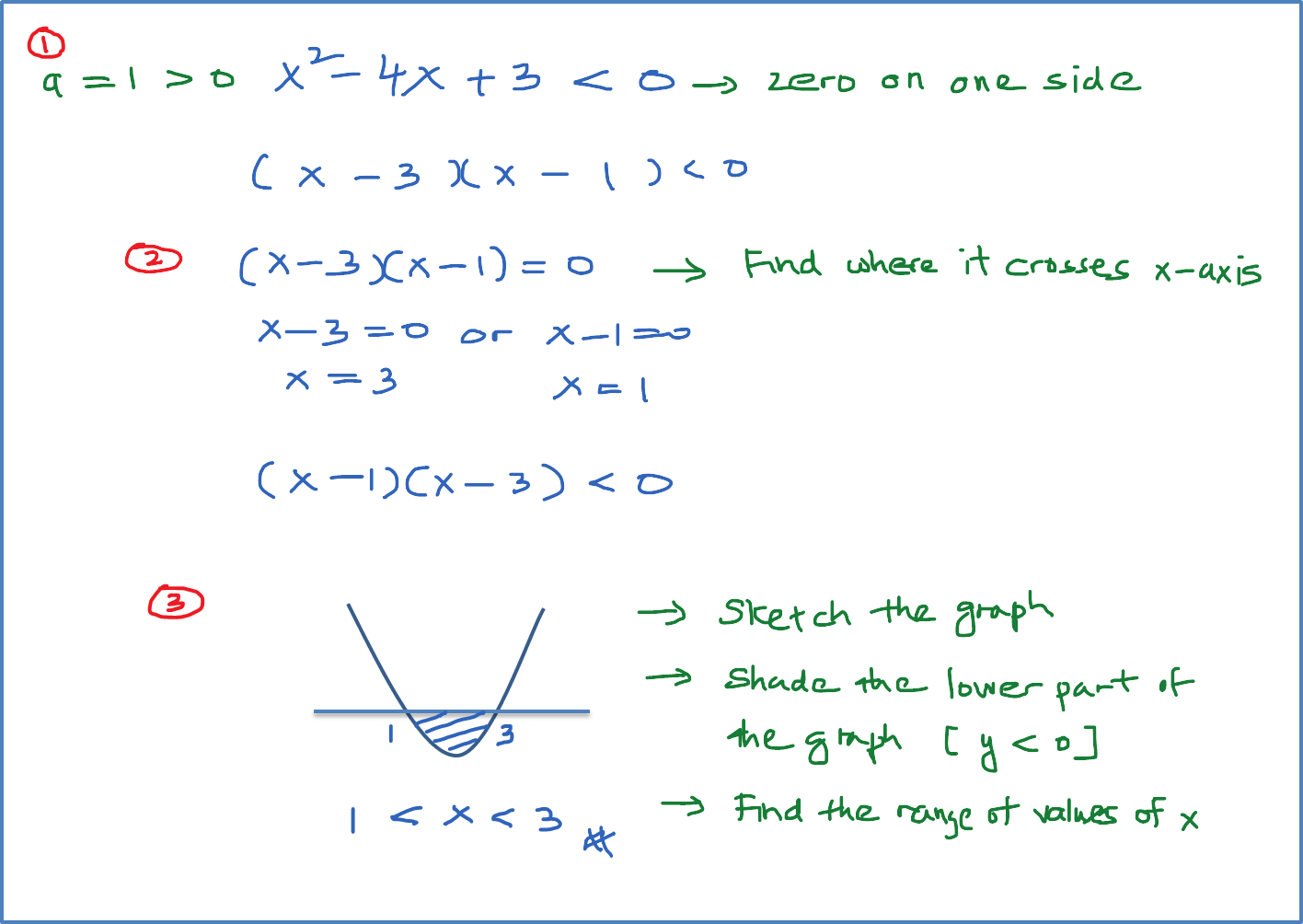
A quadratic inequality is an expression that sets a quadratic function (of the form ax² + bx + c) in relation to a value using inequality symbols such as <, >, ≤, or ≥. The solution to a quadratic inequality is the range of values for the variable that satisfies the inequality. For example, the inequality x² - 5x + 6 < 0 asks for the values of x for which the quadratic expression is less than zero.

Basic Terminology

Before diving into solving quadratic inequalities, it’s essential to understand some basic terms:

• Quadratic Function: A function of the form f(x) = ax² + bx + c, where a, b, and c are constants and a ≠ 0.
• Roots/Zeros: The values of x that make the quadratic function equal to zero.
• Interval: A range of values on the number line.
• Test Point: A value chosen from an interval to determine whether the inequality holds true in that interval.

Methods to Solve Quadratic Inequalities

There are two primary methods for solving quadratic inequalities: the graphical method and the algebraic method. Each has its advantages and can be used based on the specific problem at hand.

Graphical Method

The graphical method involves plotting the quadratic function on a coordinate plane and analyzing the regions defined by the inequality. Here’s how to do it:

1. Graph the corresponding quadratic equation (ax² + bx + c = 0) to find the roots of the equation.
2. Identify the regions on the x-axis defined by the roots.
3. Determine whether the quadratic opens upwards (a > 0) or downwards (a < 0).
4. Use test points in each region to check which intervals satisfy the inequality.

Algebraic Method

The algebraic method involves manipulating the inequality algebraically to find the solution. Follow these steps:

1. Rearrange the inequality to set one side to zero (e.g., ax² + bx + c < 0 becomes ax² + bx + c = 0).
2. Solve for the roots using the quadratic formula: x = (-b ± √(b² - 4ac)) / 2a.
3. Determine the intervals created by the roots.
4. Choose test points within each interval to check which satisfy the original inequality.

Example Problems

Let’s go through a couple of examples to illustrate both methods of solving quadratic inequalities.

Example 1: Using the Graphical Method

Solve the inequality x² - 4x - 5 < 0.

1. First, find the roots by solving x² - 4x - 5 = 0. The roots are x = 5 and x = -1.
2. Next, plot the quadratic function. Since the coefficient of x² is positive, the parabola opens upwards.
3. Identify the intervals: (-∞, -1), (-1, 5), and (5, ∞).
4. Choose test points: For (-∞, -1) use x = -2, for (-1, 5) use x = 0, and for (5, ∞) use x = 6.
5. Test these points in the original inequality to find that the solution is (-1, 5).

Example 2: Using the Algebraic Method

Solve the inequality x² + 2x - 3 ≥ 0.

1. Rearrange to find the roots: x² + 2x - 3 = 0. The roots are x = 1 and x = -3.
2. Identify intervals: (-∞, -3), (-3, 1), and (1, ∞).
3. Choose test points: For (-∞, -3) use x = -4, for (-3, 1) use x = 0, and for (1, ∞) use x = 2.
4. Test these points to find that the solution is (-∞, -3] ∪ [1, ∞).

Real-World Applications

Quadratic inequalities have various applications in real life, particularly in fields such as physics, engineering, and economics. Here are a few examples:

• Projectile Motion: Quadratic inequalities can help determine the height of an object over time.
• Cost Analysis: Businesses can use quadratic inequalities to analyze profit margins and production costs.
• Optimization Problems: Quadratic inequalities are often used to find maximum and minimum values in optimization scenarios.

Common Mistakes

When solving quadratic inequalities, it’s essential to avoid common pitfalls:

• Forgetting to check all intervals after finding the roots.
• Misinterpreting the direction of the inequality when selecting test points.
• Not considering the endpoints of the intervals when dealing with ≤ or ≥ inequalities.

Tips for Solving Quadratic Inequalities

Here are some tips to help you effectively solve quadratic inequalities:

• Always graph the quadratic function to visualize the problem.
• Make sure to double-check your calculations for roots.
• Practice with various types of inequalities to build confidence.
• Use a clear and organized approach to avoid confusion.

Conclusion

In summary, solving quadratic inequalities requires an understanding of both graphical and algebraic methods. By mastering these techniques, you can unlock the ability to tackle a wide range of mathematical problems. Remember to practice regularly and refer back to this guide as needed. If you have any questions or want to share your experiences with quadratic inequalities, feel free to leave a comment below!

For more mathematical insights and tutorials, don't forget to check out our other articles. Happy learning!